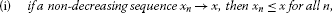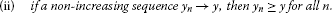Coupled coincidence point theorems for contractions in generalized fuzzy metric spaces

Abstract

In this paper, we introduce the concept of a mixed g-monotone mapping and prove coupled coincidence and common coupled fixed point theorems for mappings under ϕ-contractive conditions in partially ordered generalized fuzzy metric spaces. We also give an example to illustrate the theorems.

MSC:47H10, 54H25.

1 Introduction

The theory of fuzzy sets has evolved in many directions after investigation of the notion of fuzzy sets by Zadeh [1]. Many authors have introduced the concept of a fuzzy metric space in different ways [2, 3]. George and Veeramani [4, 5] modified the concept of a fuzzy metric space introduced by Kramosil and Michalek [3] and defined a Hausdorff topology on this fuzzy metric space. They showed also that every metric induces a fuzzy metric. Later, many fixed point theorems in fuzzy metric spaces and probabilistic metric spaces have been obtained by [6–10].

Nieto and Lopez [11], Ran and Reurings [12], Petrusel and Rus [13] presented some new results for contractions in partially ordered metric spaces. The main idea in [11, 12] involves combining the ideas of the iterative technique in the contractive mapping principle with those in the monotone technique, discussing the existence of a solution to first-order ordinary differential equations with periodic boundary conditions and some applications to linear and nonlinear matrix equations.

Bhaskar and Lakshmikantham [14], Lakshmikantham and Ćirić [15] discussed coupled coincidence and coupled fixed point theorems for two mappings F and g, where F has the mixed g-monotone property and F and g commute. The results were used to study the existence of a unique solution to a periodic boundary value problem. In [16], Choudhury and Kundu established a similar result under the condition that F and g are compatible mappings and the function g is monotone increasing. For more details on ordered metric spaces, we refer to [17–19] and references mentioned therein.

Alternatively Mustafa and Sims [20] introduced a new notion of a generalized metric space called G-metric space. Mujahid Abbas et al. [23] proved a unique fixed point of four R-weakly commuting maps in G-metric spaces, and Mujahid Abbas et al. [24] obtained some common fixed point results of maps satisfying the generalized $(\phi ,\psi )$-weak commuting condition in partially ordered G-metric spaces. Rao et al. [22] proved two unique common coupled fixed-point theorems for three mappings in symmetric G-fuzzy metric spaces. Sun and Yang [21] introduced the concept of G-fuzzy metric spaces and proved two common fixed-point theorems for four mappings. Some interesting references on G-metric spaces are [22–25].

In this paper, we introduce the concept of a mixed g-monotone mapping, which is a generalization of the mixed monotone mapping, and prove coupled coincidence point and coupled common fixed point theorems for mappings under ϕ-contractive conditions in partially ordered G-fuzzy metric spaces. The work is an extension of the fixed point result in fuzzy metric spaces and the condition is different from [14–16] even in metric spaces. We also give an example to illustrate the theorems.

Recall that if $(X,\le )$ is a partially ordered set and $F:X\to X$ satisfies that for $x,y\in X$, $x\le y$ implies $F(x)\le F(y)$, then a mapping F is said to be non-decreasing. Similarly, a non-increasing mapping is defined.

Before giving our main results, we recall some of the basic concepts and results in G-metric spaces and G-fuzzy metric spaces.

2 Preliminaries

Definition 2.1 [20]

Let X be a nonempty set, and let $G:X\times X\times X\to [0,+\mathrm{\infty })$ be a function satisfying the following properties:

(G-1) $G(x,y,z)=0$ if $x=y=z$,

(G-2) $0<G(x,x,y)$ for all $x,y\in X$ with $x\ne y$,

(G-3) $G(x,x,y)\le G(x,y,z)$ for all $x,y,z\in X$ with $y\ne z$,

(G-4) $G(x,y,z)=G(x,z,y)=G(y,z,x)=\cdots$, symmetry in all three variables,

(G-5) $G(x,y,z)\le G(x,a,a)+G(a,y,z)$ for all $x,y,z,a\in X$.

The function G is called a generalized metric or a G-metric on X and the pair $(X,G)$ is called a G-metric space.

Definition 2.2 [20]

The G-metric space $(X,G)$ is called symmetric if $G(x,x,y)=G(x,y,y)$ for all $x,y\in X$.

Definition 2.3 [20]

Let $(X,G)$ be a G-metric space, and let $\{x_n\}$ be a sequence in X. A point $x\in X$ is said to be the limit of $\{x_n\}$ if and only if ${lim}_{n,m\to \mathrm{\infty }}G(x,x_n,x_m)=0$. In this case, the sequence $\{x_n\}$ is said to be G-convergent to x.

Definition 2.4 [20]

Let $(X,G)$ be a G-metric space, and let $\{x_n\}$ be a sequence in X. $\{x_n\}$ is called a G-Cauchy sequence if and only if ${lim}_{n,m,l\to \mathrm{\infty }}G(x_n,x_m,x_l)=0$. $(X,G)$ is called G-complete if every G-Cauchy sequence in $(X,G)$ is G-convergent in $(X,G)$.

Proposition 2.1 [20]

In a G-metric space $(X,G)$, the following are equivalent:

• (i) The sequence $\{x_n\}$ is G-Cauchy.

• (ii) For every $\epsilon >0$, there exists $N\in \mathbb{N}$ such that $G(x_n,x_m,x_m)<\epsilon$ for all $n,m\ge N$.

Proposition 2.2 [20]

Let $(X,G)$ be a G-metric space; then the function $G(x,y,z)$ is jointly continuous in all three of its variables.

Proposition 2.3 [20]

Let $(X,G)$ be a G-metric space; then for any $x,y,z,a\in X$, it follows that

• (i) if $G(x,y,z)=0$, then $x=y=z$,

• (ii) $G(x,y,z)\le G(x,x,y)+G(x,x,z)$,

• (iii) $G(x,y,y)\le 2G(x,x,y)$,

• (iv) $G(x,y,z)\le G(x,a,z)+G(a,y,z)$,

• (v) $G(x,y,z)\le \frac{2}{3}(G(x,a,a)+G(y,a,a)+G(z,a,a))$.

Let $(X,d)$ be a metric space. One can verify that $(X,G)$ is a G-metric space, where

$$G(x,y,z)=max\{d(x,y),d(y,z),d(z,x)\}$$

or

$$G(x,y,z)=\frac{1}{3}(d(x,y)+d(y,z)+d(z,x)).$$

If $(X,G)$ is a G-metric space, it easy to verify that $(X,d_G)$ is a metric space, where $d_G(x,y)=\frac{1}{2}(G(x,x,y)+G(x,y,y))$.

Definition 2.5 [26]

A binary operation $\ast :[0,1]\times [0,1]\to [0,1]$ is a continuous t-norm if ∗ satisfies the following conditions:

• (i) ∗ is commutative and associative;

• (ii) ∗ is continuous;

• (iii) $a\ast 1=a$ for all $a\in [0,1]$;

• (iv) $a\ast b\le c\ast d$ whenever $a\le c$ and $b\le d$ for all $a,b,c,d\in [0,1]$.

Definition 2.6 [21]

A 3-tuple $(X,G,\ast )$ is said to be a G-fuzzy metric space (denoted by GF space) if X is an arbitrary nonempty set, ∗ is a continuous t-norm and G is a fuzzy set on $X^3\times (0,+\mathrm{\infty })$ satisfying the following conditions for each $t,s>0$:

(GF-1) $G(x,x,y,t)>0$ for all $x,y\in X$ with $x\ne y$;

(GF-2) $G(x,x,y,t)\ge G(x,y,z,t)$ for all $x,y,z\in X$ with $y\ne z$;

(GF-3) $G(x,y,z,t)=1$ if and only if $x=y=z$;

(GF-4) $G(x,y,z,t)=G(p(x,y,z),t)$, where p is a permutation function;

(GF-5) $G(x,a,a,t)\ast G(a,y,z,s)\le G(x,y,z,t+s)$ (the triangle inequality);

(GF-6) $G(x,y,z,\cdot ):(0,\mathrm{\infty })\to [0,1]$ is continuous.

Remark 2.1 Let $x=w$, $y=u$, $z=u$, $a=v$ in (GF-5), we have

$$G(w,u,u,t+s)\ge G(w,v,v,t)\ast G(v,u,u,s),$$

which implies that

$$G(u,u,w,s+t)\ge G(u,u,v,s)\ast G(v,v,w,t),$$

for all $u,v,w\in X$ and $s,t>0$.

A GF space is said to be symmetric if $G(x,x,y,t)=G(x,y,y,t)$ for all $x,y\in X$ and for each $t>0$.

Example 2.1 Let X be a nonempty set, and let G be a G-metric on X. Define the t-norm $a\ast b=min\{a,b\}$ and for all $x,y,z\in X$ and $t>0$, $G(x,y,z,t)=\frac{t}{t+G(x,y,z)}$. Then $(X,G,\ast )$ is a GF space.

Remark 2.2 If $(X,M,\ast )$ is a fuzzy metric space [4], then $(X,G,\ast )$ is a GF space, where

$$G(x,y,z,t)=min\{M(x,y,t),M(y,z,t),M(z,x,t)\}.$$

In fact, we only need to verify (GF-5). Since

we have

which implies that (GF-5) holds.

Remark 2.3 If $(X,G,\ast )$ is a symmetric GF space, let $M(x,y,t)=G(x,y,y,t)$, then $(X,M,\ast )$ is a fuzzy metric space [4].

Let $(X,G,\ast )$ be a GF space. For $t>0$, the open ball $B_G(x,r,t)$ with center $x\in X$ and radius $0<r<1$ is defined by

$$B_G(x,r,t)=\{y\in X:G(x,y,y,t)>1-r\}.$$

A subset $A\subset X$ is called an open set if for each $x\in A$, there exist $t>0$ and $0<r<1$ such that $B_G(x,r,t)\subset A$.

Definition 2.7 [21]

Let $(X,G,\ast )$ be a GF space, then

• (1) a sequence $\{x_n\}$ in X is said to be convergent to x (denoted by ${lim}_{n\to \mathrm{\infty }}{x}_n=x$) if

  $$\underset{n\to \mathrm{\infty }}{lim}G(x_n,x_n,x,t)=1$$

for all $t>0$.

• (2) a sequence $\{x_n\}$ in X is said to be a Cauchy sequence if

  $$\underset{n,m\to \mathrm{\infty }}{lim}G(x_n,x_n,x_m,t)=1,$$

that is, for any $\epsilon >0$ and for each $t>0$, there exists $n_0\in \mathbb{N}$ such that

$$G(x_n,x_n,x_m,t)>1-\epsilon ,$$

for $n,m\ge n_0$.

• (3) A GF space $(X,G,\ast )$ is said to be complete if every Cauchy sequence in X is convergent.

Lemma 2.1 [21]

Let $(X,G,\ast )$ be a GF space. Then $G(x,y,z,t)$ is non-decreasing with respect to t for all $x,y,z\in X$.

Lemma 2.2 [21]

Let $(X,G,\ast )$ be a GF space. Then G is a continuous function on $X^3\times (0,+\mathrm{\infty })$.

In the rest of the paper, $(X,G,\ast )$ will denote a GF space with a continuous t-norm ∗ defined as $a\ast b=min\{a,b\}$ for all $a,b\in [0,1]$, and we assume that

$$\underset{t\to \mathrm{\infty }}{lim}G(x,y,z,t)=1,\mathrm{\forall }x,y,z\in X.$$

(P)

Define $\mathrm{\Phi }=\{\varphi :R^{+}\to R^{+}\}$, where $R^{+}=[0,+\mathrm{\infty })$ and each $\varphi \in \mathrm{\Phi }$ satisfies the following conditions:

(Φ-1) ϕ is strict increasing;

(Φ-2) ϕ is upper semi-continuous from the right;

(Φ-3) ${\sum }_{n=0}^{\mathrm{\infty }}{\varphi }^n(t)<+\mathrm{\infty }$ for all $t>0$, where ${\varphi }^{n+1}(t)=\varphi ({\varphi }^n(t))$.

Let ${\varphi }_1(t)=\frac{t}{t+1}$, ${\varphi }_2(t)=kt$, where $0<k<1$, then ${\varphi }_1,{\varphi }_2\in \mathrm{\Phi }$.

It is easy to prove that if $\varphi \in \mathrm{\Phi }$, then $\varphi (t)<t$ for all $t>0$.

Using (P), one can prove the following lemma.

Lemma 2.3 Let $(X,G,\ast )$ be a GF space. If there exists $\varphi \in \mathrm{\Phi }$ such that if $G(x,y,z,\varphi (t))\ge G(x,y,z,t)$ for all $t>0$, then $x=y=z$.

Lemma 2.4 Let $(X,G,\ast )$ be a GF space. If we define $E_{\lambda }:X\times X\times X\to [0,\mathrm{\infty })$ by

$$E_{\lambda }(x,y,z)=inf\{t>0,G(x,y,z,t)>1-\lambda \}$$

(2.1)

for all $\lambda \in (0,1]$ and $x,y,z\in X$, then we have:

• (1) for each $\lambda \in (0,1]$, there exists $\mu \in (0,1]$ such that

  $$E_{\lambda }(x_1,x_1,x_n)\le \sum _{i=1}^{n-1}{E}_{\mu }(x_i,x_i,x_{i+1}),\mathrm{\forall }{x}_1,\dots ,x_n\in X.$$

• (2) The sequence ${\{x_n\}}_{n\in \mathbb{N}}$ in X is convergent if and only if $E_{\lambda }(x_n,x_n,x)\to 0$ as $n\to \mathrm{\infty }$ for all $\lambda \in (0,1]$.

Proof (1) For any $\lambda \in (0,1]$, let $\mu \in (0,1]$ and $\mu <\lambda$, and so, by the triangular inequality (GF-5) and Remark 2.1, for any $\delta >0$, we have

which implies, by Definition 2.1 of $E_{\mu }$, that

$$E_{\lambda }(x_1,x_1,x_n)\le E_{\mu }(x_1,x_1,x_2)+E_{\mu }(x_2,x_2,x_3)+\cdot \cdot \cdot +E_{\mu }(x_{n-1},x_{n-1},x_n)+(n-1)\delta .$$

Since $\delta >0$ is arbitrary, we have

$$E_{\lambda }(x_1,x_1,x_n)\le E_{\mu }(x_1,x_1,x_2)+E_{\mu }(x_2,x_2,x_3)+\cdot \cdot \cdot +E_{\mu }(x_{n-1},x_{n-1},x_n).$$

• (2) Since G is continuous in its fourth argument, by Definition 2.1 of $E_{\mu }$, we have

  $$G(x_n,x_n,x,\eta )>1-\lambda \text{for all }\eta >0.$$

This proved the lemma. □

Lemma 2.5 Let $(X,G,\ast )$ be a GF space and $\{y_n\}$ be a sequence in X. If there exists $\varphi \in \mathrm{\Phi }$ such that

$$G(y_n,y_n,y_{n+1},\varphi (t))\ge G(y_{n-1},y_{n-1},y_n,t)\ast G(y_n,y_n,y_{n+1},t)$$

(2.2)

for all $t>0$ and $n=1,2,\dots$, then $\{y_n\}$ is a Cauchy sequence in X.

Proof Let ${\{E_{\lambda }(x,y,z)\}}_{\lambda \in (0,1]}$ be defined by (2.1). For each $\lambda \in (0,1]$ and $n\in \mathbb{N}$, putting $a_n=E_{\lambda }(y_{n-1},y_{n-1},y_n)$, we will prove that

$$a_{n+1}\le \varphi (a_n),\mathrm{\forall }n\in \mathbb{N}.$$

(2.3)

Since ϕ is upper semi-continuous from right, for given $\epsilon >0$ and each $a_n$, there exists $p_n>a_n$ such that $\varphi (p_n)<\varphi (a_n)+\epsilon$. From the definition of $E_{\lambda }$ by (2.1), it follows from $p_n>a_n=E_{\lambda }(y_{n-1},y_{n-1},y_n)$ that $G(y_{n-1},y_{n-1},y_n,p_n)>1-\lambda$ for all $n\in \mathbb{N}$.

Thus, by (2.2), (2.3) and Lemma 2.1, we get

Again by Definition 2.1, we get

$$\begin{array}{rcl}{E}_{\lambda }(y_n,y_n,y_{n+1})& \le & \varphi (max\{p_n,p_{n+1}\})=max\{\varphi (p_n),\varphi (p_{n+1})\}\\ \le & max\{\varphi (a_n),\varphi (a_{n+1})\}+\epsilon .\end{array}$$

By the arbitrariness of ε, we have

$$a_{n+1}=E_{\lambda }(y_n,y_n,y_{n+1})\le max\{\varphi (a_n),\varphi (a_{n+1})\}.$$

(2.4)

So, we can infer that $a_{n+1}\le \varphi (a_n)$. If not, then by (2.4), we have $a_{n+1}\le \varphi (a_{n+1})<a_{n+1}$, which is a contradiction. Hence, (2.4) implies that $a_{n+1}\le \varphi (a_n)$, and (2.3) is proved.

Again and again using (2.3), we get

$$E_{\lambda }(y_n,y_n,y_{n+1})\le \varphi (E_{\lambda }(y_{n-1},y_{n-1},y_n))\le \cdot \cdot \cdot \le {\varphi }^n(E_{\lambda }(y_0,y_0,y_1))\text{for all }n\in \mathbb{N}.$$

By Lemma 2.4, for each $\lambda \in (0,1]$, there exists $\mu \in (0,\lambda ]$ such that

$$E_{\lambda }(y_n,y_n,y_m)\le \sum _{i=n}^{m-1}{E}_{\mu }(y_i,y_i,y_{i+1}),\mathrm{\forall }m,n\in \mathbb{N}\text{ with }m>n.$$

(2.5)

Since $\varphi \in \mathrm{\Phi }$, by condition (Φ-3) we have ${\sum }_{n=0}^{\mathrm{\infty }}{\varphi }^n(E_{\mu }(y_0,y_0,y_1))<+\mathrm{\infty }$. So, for given $\epsilon >0$, there exists $n_0\in \mathbb{N}$ such that ${\sum }_{i=n_0}^{\mathrm{\infty }}{\varphi }^i(E_{\mu }(y_0,y_0,y_1))<\epsilon$. Thus, it follows from (2.5) that

$$E_{\lambda }(y_n,y_n,y_m)\le \sum _{i=n}^{\mathrm{\infty }}{\varphi }^i(E_{\mu }(y_0,y_0,y_1))<\epsilon ,\mathrm{\forall }n\ge n_0,$$

which implies that $G(y_n,y_n,y_m,\epsilon )>1-\lambda$ for all $m,n\in \mathbb{N}$ with $m>n\ge n_0$. Therefore, $\{y_n\}$ is a Cauchy sequence in X. □

3 Main results

Definition 3.1 [14]

Let $(X,\le )$ be a partially ordered set. The mapping F is said to have the mixed monotone property if F is monotone non-decreasing in its first argument and is monotone non-increasing in its second argument; that is, for any $x,y\in X$,

$$x_1,x_2\in X,x_1\le x_2\Rightarrow F(x_1,y)\le F(x_2,y),$$

(3.1)

and

$$y_1,y_2\in X,y_1\le y_2\Rightarrow F(x,y_1)\ge F(x,y_2).$$

(3.2)

Definition 3.2 [14]

An element $(x,y)\in X\times X$ is called a coupled fixed point of the mapping $F:X\times X$ if

$$F(x,y)=x,F(y,x)=y.$$

Definition 3.3 [15]

Let $(X,\le )$ be a partially ordered set and $F:X\times X\to X$ and $g:X\to X$. We say F has the mixed g-monotone property if F is monotone g-non-decreasing in its first argument and is monotone g-non-increasing in its second argument; that is, for any $x,y\in X$,

(3.3)

and

(3.4)

Note that if g is the identity mapping, then Definition 3.3 reduces to Definition 3.1.

Example 3.1 Let $X=[-1,1]$ with the natural ordering of real numbers. Let $g:X\to X$ and $F:X\times X\to X$ be defined as

$$g(x)=x^4,F(x,y)=x^2-y^2.$$

Then F is not mixed monotone but mixed g-monotone.

Definition 3.4 [15]

Let X be a nonempty set, $F:X\times X\to X$ and $g:X\to X$, then

• (1) An element $(x,y)\in X\times X$ is called a coupled coincidence point of the mappings F and g if

  $$F(x,y)=g(x),F(y,x)=g(y).$$

• (2) An element $(x,y)\in X\times X$ is called a common coupled fixed point of the mappings F and g if

  $$F(x,y)=g(x)=x,F(y,x)=g(y)=y.$$

Definition 3.5 The mappings $F:X\times X\to X$ and $g:X\to X$ are said to be compatible if

$$\underset{n\to \mathrm{\infty }}{lim}G(gF(x_n,y_n),gF(x_n,y_n),F(g(x_n),g(y_n)),t)=1$$

and

$$\underset{n\to \mathrm{\infty }}{lim}G(gF(y_n,x_n),gF(y_n,x_n),F(g(y_n),g(x_n)),t)=1$$

for all $t>0$ whenever $\{x_n\}$ and $\{y_n\}$ are sequences in X such that

$$\underset{n\to \mathrm{\infty }}{lim}F(x_n,y_n)=\underset{n\to \mathrm{\infty }}{lim}g(x_n)=x,\underset{n\to \mathrm{\infty }}{lim}F(y_n,x_n)=\underset{n\to \mathrm{\infty }}{lim}g(y_n)=y$$

for all $x,y\in X$ are satisfied.

Definition 3.6 [16]

The mappings $F:X\times X\to X$ and $g:X\to X$ are called w-compatible if

$$g(F(x,y))=F(gx,gy),g(F(y,x))=F(gy,gx)$$

whenever $g(x)=F(x,y)$ and $g(y)=F(y,x)$ for some $(x,y)\in X\times X$.

Remark 3.1 It is easy to prove that if F and g are compatible then they are w-compatible.

Theorem 3.1 Let $(X,\le )$ be a partially ordered set and $(X,G,\ast )$ be a complete GF space. Let $F:X\times X\to X$ and $g:X\to X$ be two mappings such that F has the mixed g-monotone property and there exists $\varphi \in \mathrm{\Phi }$ such that

(3.5)

for all $x,y,u,v\in X$, $t>0$ for which $g(x)\le g(u)$ and $g(y)\ge g(v)$, or $g(x)\ge g(u)$ and $g(y)\le g(v)$.

Suppose $F(X\times X)\subseteq g(X)$, g is continuous and F and g are compatible. Also suppose

• (a) F is continuous or

• (b) X has the following properties:

  

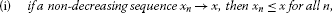  (3.6)
  

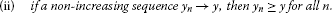  (3.7)

If there exists $x_0,y_0\in X$ such that $g(x_0)\le F(x_0,y_0)$ and $g(y_0)\ge F(y_0,x_0)$, then there exist $x,y\in X$ such that $g(x)=F(x,y)$ and $g(y)=F(y,x)$; that is, F and g have a coupled coincidence point in X.

Proof Let $x_0,y_0\in X$ be such that $g(x_0)\le F(x_0,y_0)$ and $g(y_0)\ge F(y_0,x_0)$. Since $F(X\times X)\subseteq g(X)$, we can choose $x_1,y_1\in X$ such that $g(x_1)=F(x_0,y_0)$ and $g(y_1)=F(y_0,x_0)$. Continuing in this way, we construct two sequences $\{x_n\}$ and $\{y_n\}$ in X such that

$$g(x_{n+1})=F(x_n,y_n),g(y_{n+1})=F(y_n,x_n),\text{for all }n\ge 0.$$

(3.8)

We shall show that

(3.9)

(3.10)

for all $n\ge 0$.

We shall use the mathematical induction. Let $n=0$. Since $g(x_0)\le F(x_0,y_0)$ and $g(y_0)\ge F(y_0,x_0)$, and as $g(x_1)=F(x_0,y_0)$ and $g(y_1)=F(y_0,x_0)$, we have $g(x_0)\le g(x_1)$ and $g(y_0)\ge g(y_1)$. Thus, (3.9) and (3.10) hold for $n=0$. Suppose now that (3.9) and (3.10) hold for some fixed $n\ge 0$. Then since $g(x_n)\le g(x_{n+1})$ and $g(y_n)\ge g(y_{n+1})$, and as F has the mixed g-monotone property, from (3.8) and (3.3),

$$\begin{array}{l}g(x_{n+1})=F(x_n,y_n)\le F(x_{n+1},y_n),\\ F(y_{n+1},x_n)\le F(y_n,x_n)=g(y_{n+1}),\end{array}\}$$

(3.11)

and from (3.8) and (3.4),

$$\begin{array}{l}g(x_{n+2})=F(x_{n+1},y_{n+1})\ge F(x_{n+1},y_n),\\ F(y_{n+1},x_n)\ge F(y_{n+1},x_{n+1})=g(y_{n+2}).\end{array}\}$$

(3.12)

Now from (3.11) and (3.12), we get $g(x_{n+1})\le g(x_{n+2})$ and $g(y_{n+1})\ge g(y_{n+2})$. Thus, by mathematical induction, we conclude that (3.9) and (3.10) hold for all $n\ge 0$. Therefore,

$$g(x_0)\le g(x_1)\le g(x_2)\le \cdots \le g(x_n)\le g(x_{n+1})\le \cdots$$

(3.13)

and

$$g(y_0)\ge g(y_1)\ge g(y_2)\ge \cdots \ge g(y_n)\ge g(y_{n+1})\ge \cdots .$$

(3.14)

By putting ($x=x_{n-1}$, $y=y_{n-1}$, $u=x_n$, $v=y_n$) in (3.5), we get

So, by (3.8), we have

Now, by Lemma 2.5, $\{g(x_n)\}$ is a Cauchy sequence.

By putting ($x=y_n$, $y=x_n$, $u=y_{n-1}$, $v=x_{n-1}$) in (3.5), we get

So, by (3.8), we have

Now, by Lemma 2.5, $\{g(y_n)\}$ is also a Cauchy sequence.

Since X is complete, there exist $x,y\in X$ such that

$$\underset{n\to \mathrm{\infty }}{lim}F(x_n,y_n)=\underset{n\to \mathrm{\infty }}{lim}g(x_n)=x,\underset{n\to \mathrm{\infty }}{lim}F(y_n,x_n)=\underset{n\to \mathrm{\infty }}{lim}g(y_n)=y.$$

(3.15)

Since F and g are compatible, we have by (3.15)

$$\underset{n\to \mathrm{\infty }}{lim}G(g(F(x_n,y_n)),g(F(x_n,y_n)),F(g(x_n),g(y_n)),t)=1$$

(3.16)

and

$$\underset{n\to \mathrm{\infty }}{lim}G(g(F(y_n,x_n)),g(F(y_n,x_n)),F(g(y_n),g(x_n)),t)=1$$

(3.17)

for all $t>0$. Next, we prove that $g(x)=F(x,y)$ and $g(y)=F(y,x)$.

Let (a) hold. Since F and g are continuous, by Lemma 2.2, taking limits as $n\to \mathrm{\infty }$ in (3.16) and (3.17), we get

$$G(g(x),g(x),F(x,y),t)=1,G(g(y),g(y),F(y,x),t)=1$$

for all $t>0$. We have $g(x)=F(x,y)$, $g(y)=F(y,x)$.

Next, we suppose that (b) holds. By (3.9), (3.10), (3.15), we have for all $n\ge 0$

$$g(x_n)\le x,g(y_n)\ge y.$$

(3.18)

Since F and g are compatible and g is continuous, by (3.16) and (3.17), we have

$$\underset{n\to \mathrm{\infty }}{lim}g(g{x}_n)=gx=\underset{n\to \mathrm{\infty }}{lim}g(F(x_n,y_n))=\underset{n\to \mathrm{\infty }}{lim}F(g(x_n),g(y_n))$$

(3.19)

and

$$\underset{n\to \mathrm{\infty }}{lim}g(g{y}_n)=gy=\underset{n\to \mathrm{\infty }}{lim}g(F(y_n,x_n))=\underset{n\to \mathrm{\infty }}{lim}F(g(y_n),g(x_n)).$$

(3.20)

Now, we have

$$\begin{array}{rcl}G(gx,gx,F(x,y),\varphi (t))& \ge & G(gx,gx,g(g{x}_{n+1}),\varphi (t)-\varphi (kt))\\ \ast G(g(g{x}_{n+1}),g(g{x}_{n+1}),F(x,y),\varphi (kt))\end{array}$$

for all $0\le k<1$. Taking the limit as $n\to \mathrm{\infty }$ in the above inequality, by continuity of G, using (3.8) and (3.19), we have

By (3.5), (3.19) and the above inequality, we have that

Letting $k\to 1$, which implies that $gx=F(x,y)$ by Lemma 2.3, and similarly, by the virtue of (3.8), (3.15) and (3.20), we get $gy=F(y,x)$. Thus, we have proved that F and g have a coupled coincidence point in X.

This completes the proof of Theorem 3.1. □

Taking $g=I$ (the identity mapping) in Theorem 3.1, we get the following consequence.

Corollary 3.1 Let $(X,\le )$ be a partially ordered set and $(X,G,\ast )$ be a complete GF space. Let $F:X\times X\to X$ be a mapping such that F has the mixed monotone property and there exists $\varphi \in \mathrm{\Phi }$ such that

$$G(F(x,y),F(x,y),F(u,v),\varphi (t))\ge G(x,x,u,t)\ast G(x,x,F(x,y),t)\ast G(u,u,F(u,v),t)$$

for all $x,y,u,v\in X$, $t>0$ for which $x\le u$ and $y\ge v$. Suppose

• (a) F is continuous or

• (b) X has the following properties:

• (i) if a non-decreasing sequence $x_n\to x$, then $x_n\le x$ for all n,

• (ii) if a non-increasing sequence $y_n\to y$, then $y_n\ge y$ for all n.

If there exists $x_0,y_0\in X$ such that $x_0\le F(x_0,y_0)$ and $y_0\ge F(y_0,x_0)$, then there exist $x,y\in X$ such that $x=F(x,y)$ and $y=F(y,x)$; that is, F has a coupled fixed point in X.

Now, we shall prove the existence and uniqueness theorem of a coupled common fixed point. Note that if $(S,\le )$ is a partially ordered set, then we endow the product $S\times S$ with the following partial order:

$$\mathit{\text{for}}(x,y),(u,v)\in S\times S,(x,y)\le (u,v)\iff x\le u,y\ge v.$$

Theorem 3.2 In addition to the hypotheses of Theorem  3.1, suppose that for every $(x,y),(x^{\star },y^{\star })\in X\times X$, there exists a $(u,v)\in X\times X$ satisfying $g(u)\le g(v)$ or $g(v)\le g(u)$ such that $(F(u,v),F(v,u))\in X\times X$ is comparable to $(F(x,y),F(y,x))$, $(F(x^{\star },y^{\star }),F(y^{\star },x^{\star }))$. Then F and g have a unique common coupled fixed point; that is, there exists a unique $(x,y)\in X\times X$ such that

$$x=g(x)=F(x,y),y=g(y)=F(y,x).$$

Proof From Theorem 3.1, the set of coupled coincidence points is nonempty. We shall show that if $(x,y)$ and $(x^{\star },y^{\star })$ are coupled coincidence points, that is, if $g(x)=F(x,y)$, $g(y)=F(y,x)$ and $g(x^{\star })=F(x^{\star },y^{\star })$, $g(y^{\star })=F(y^{\star },x^{\star })$, then

$$g(x)=g\left(x^{\star }\right),g(y)=g\left(y^{\star }\right).$$

(3.21)

By assumption, there is $(u,v)\in X\times X$ such that $(F(u,v),F(v,u))$ is comparable with $(F(x,y),F(y,x))$, $(F(x^{\star },y^{\star }),F(y^{\star },x^{\star }))$. Put $u_0=u$, $v_0=v$ and choose $u_1,v_1\in X$ so that $g(u_1)=F(u_0,v_0)$ and $g(v_1)=F(v_0,u_0)$. Then, similarly as in the proof of Theorem 3.1, we can inductively define sequences $\{g(u_n)\}$ and $\{g(v_n)\}$ such that

$$g(u_{n+1})=F(u_n,v_n),g(v_{n+1})=F(v_n,u_n).$$

With the similar proof as in Theorem 3.1, we can prove that the limits of $\{g(u_n)\}$ and $\{g(v_n)\}$ exist.

Since $(F(x,y),F(y,x))=(g(x_1),g(y_1))=(g(x),g(y))$ and $(F(u,v),F(v,u))=(g(u_1),g(v_1))$ are comparable, it is easy to show that $(g(x),g(y))$ and $(g(u_n),g(v_n))$ are comparable for all $n\ge 1$. Thus, from (3.5),

for each $n\ge 1$. Letting $n\to \mathrm{\infty }$, we get

$$\underset{n\to \mathrm{\infty }}{lim}g(u_n)=g(x),\underset{n\to \mathrm{\infty }}{lim}g(v_n)=g(y).$$

(3.22)

Similarly, one can prove that

$$\underset{n\to \mathrm{\infty }}{lim}g(u_n)=g\left(x^{\star }\right),\underset{n\to \mathrm{\infty }}{lim}g(v_n)=g\left(y^{\star }\right).$$

(3.23)

By (3.22) and (3.23), we have

$$G(gx,gx,g{x}^{\star },t)\ge G(gx,gx,g{u}_{n+1},\frac{t}{2})\ast G(g{u}_{n+1},g{u}_{n+1},g{x}^{\star },\frac{t}{2})\to 1(n\to \mathrm{\infty }),$$

which shows that $g(x)=g(x^{\star })$.

Similarly, one can prove that $g(y)=g(y^{\star })$. Thus, we proved (3.21).

Since $g(x)=F(x,y)$ and $g(y)=F(y,x)$, by the compatibility of F and g, we can get the w-compatibility of F and g, which implies

$$g(g(x))=g(F(x,y))=F(g(x),g(y)),$$

(3.24)

and

$$g(g(y))=g(F(y,x))=F(g(y),g(x)).$$

(3.25)

Denote $g(x)=z$, $g(y)=w$. Then from (3.24) and (3.25),

$$g(z)=F(z,w),g(w)=F(w,z).$$

(3.26)

Thus, $(z,w)$ is a coupled coincidence point. From (3.21) with $x^{\star }=z$, $y^{\star }=w$, it also follows $g(z)=g(x)$, $g(w)=g(y)$, that is,

$$g(z)=z,g(w)=w.$$

(3.27)

From (3.26) and (3.27), we get

$$z=g(z)=F(z,w),w=g(w)=F(w,z).$$

Therefore, $(z,w)$ is a common coupled fixed point of F and g. To prove the uniqueness, assume that $(p,q)$ is another coupled common fixed point. Then by (3.21) we have $p=g(p)=g(z)=z$ and $q=g(q)=g(w)=w$. □

From Remark 2.3, let $(X,G,\ast )$ be a symmetric GF space. From Theorem 3.1, we get the following

Corollary 3.2 Let $(X,\le )$ be a partially ordered set and $(X,F,\ast )$ be a complete fuzzy metric space. Let $F:X\times X\to X$ and $g:X\to X$ be two mappings such that F has the mixed g-monotone property and there exists $\varphi \in \mathrm{\Phi }$ such that

$$M(F(x,y),F(u,v),\varphi (t))\ge M(gx,gu,t)\ast M(gx,F(x,y),t)\ast M(gu,F(u,v),t)$$

for all $x,y,u,v\in X$, $t>0$, for which $g(x)\le g(u)$ and $g(y)\ge g(v)$, or $g(x)\ge g(u)$ and $g(y)\le g(v)$.

Suppose $F(X\times X)\subseteq g(X)$, g is continuous and F and g are compatible. Also suppose

• (a) F is continuous or

• (b) X has the following properties:

• (i) if a non-decreasing sequence $x_n\to x$, then $x_n\le x$ for all n,

• (ii) if a non-increasing sequence $y_n\to y$, then $y_n\ge y$ for all n.

If there exist $x_0,y_0\in X$ such that $g(x_0)\le F(x_0,y_0)$ and $g(y_0)\ge F(y_0,x_0)$, then there exist $x,y\in X$ such that $g(x)=F(x,y)$ and $g(y)=F(y,x)$, that is, F and g have a coupled coincidence point in X.

Remark 3.2 Compared with the results in [15, 16], we can find that Theorem 3.1 is different in the following aspects:

• (1) We assume that F and g are compatible, which is weaker than the conditions in [15, 16], where Theorem 2.1 in [15] assumes commutation for F and g, and Theorem 3.1 in [16] requires g to be a monotone function.

(2) We have a different contractive condition from [15, 16] even in a metric space.

• (3) In our paper, we assume that $\varphi \in \mathrm{\Phi }$, which is a stronger condition than that in [15, 16]. But we would like to point out that in the case of $\varphi (t)=kt$, where $0<k<1$, the two conditions are equivalent.

Next, we give an example to demonstrate Theorem 3.1.

Example 3.2 Let $X=[0,1]$, $a\ast b=min\{a,b\}$. Then $(X,\le )$ is a partially ordered set with the natural ordering of real numbers. Let

$$G(x,y,z,t)=\frac{t}{t+|x-y|+|y-z|+|z-x|}$$

for all $x,y,z\in [0,1]$. Then $(X,G,\ast )$ is a complete GF space.

Let $g:X\to X$ and $F:X\times X\to X$ be defined as

F obeys the mixed g-monotone property.

Let $\varphi (t)=\frac{t}{3}$ for $t\in [0,\mathrm{\infty })$. Let $\{x_n\}$ and $\{y_n\}$ be two sequences in X such that

$$\underset{n\to \mathrm{\infty }}{lim}F(x_n,y_n)=a,\underset{n\to \mathrm{\infty }}{lim}g(x_n)=a,\underset{n\to \mathrm{\infty }}{lim}F(y_n,x_n)=b,\underset{n\to \mathrm{\infty }}{lim}g(y_n)=b,$$

then $a=0$, $b=0$. Now, for all $n\ge 0$,

and

$$F(y_n,x_n)=\{\begin{array}{cc}\frac{y_n^2-x_n^2}{3},\hfill & \text{if }{y}_n\ge x_n,\hfill \\ 0,\hfill & \text{if }{y}_n<x_n.\hfill \end{array}$$

Then it follows that

Hence, the mappings F and g are compatible in X. Also, $x_0=0$ and $y_0=c$ are two points in X such that

$$g(x_0)=g(0)=F(0,c)=F(x_0,y_0)$$

and

$$g(y_0)=g(c)=c^2\ge \frac{c^2}{3}=F(c,0)=F(y_0,x_0).$$

We next verify the inequality of Theorem 3.1. We take $x,y,u,v\in X$ such that $g(x)\le g(u)$ and $g(y)\ge g(v)$, that is, $x^2\le u^2$, $y^2\ge v^2$.

We consider the following cases:

Case 1: $x\ge y$ and $u\ge v$, then

$$\begin{array}{rcl}G(F(x,y),F(x,y),F(u,v),\varphi (t))& =& G(\frac{x^2-y^2}{3},\frac{x^2-y^2}{3},\frac{u^2-v^2}{3},\varphi (t))\\ =& \frac{\frac{t}{3}}{\frac{t}{3}+|\frac{(x^2-u^2)-(y^2-v^2)}{3}|}\\ =& \frac{t}{t+|(x^2-u^2)-(y^2-v^2)|}\\ \ge & \frac{t}{t+|u^2-\frac{u^2-v^2}{3}|}\\ =& G(g(u),g(u),F(u,v),t)\\ \ge & G(g(x),g(x),g(u),t)\ast G(g(x),g(x),F(x,y),t)\\ \ast G(g(u),g(u),F(u,v),t).\end{array}$$

Case 2: $x\ge y$, $u<v$. Since $x\le u$, then $u<v$ cannot happen.

Case 3: $x<y$ and $u\ge v$, then

$$\begin{array}{rcl}G(F(x,y),F(x,y),F(u,v),\varphi (t))& =& G(0,0,\frac{u^2-v^2}{3},\varphi (t))\\ =& \frac{\frac{t}{3}}{\frac{t}{3}+|\frac{(u^2-v^2)}{3}|}\\ =& \frac{t}{t+|u^2-v^2|}\\ \ge & \frac{t}{t+2|u^2-x^2|}\\ =& G(g(x),g(x),g(u),t)\\ \ge & G(g(x),g(x),g(u),t)\ast G(g(x),g(x),F(x,y),t)\\ \ast G(g(u),g(u),F(u,v),t).\end{array}$$

Case 4: $x<y$ and $u<v$ with $x^2\le u^2$ and $y^2\ge v^2$, then $F(x,y)=0$ and $F(u,v)=0$, that is, $G(F(x,y),F(x,y),F(u,v),\varphi (t))=0$. Obviously, (3.5) is satisfied.

Thus, it is verified that the functions F, g, ϕ satisfy all the conditions of Theorem 3.1. Here $(0,0)$ is the coupled coincidence point of F and g in X, which is also their common coupled fixed point.